Theory bites are a collection of basic hydraulic theory and will touch upon pump design and other areas of pump industry knowledge.
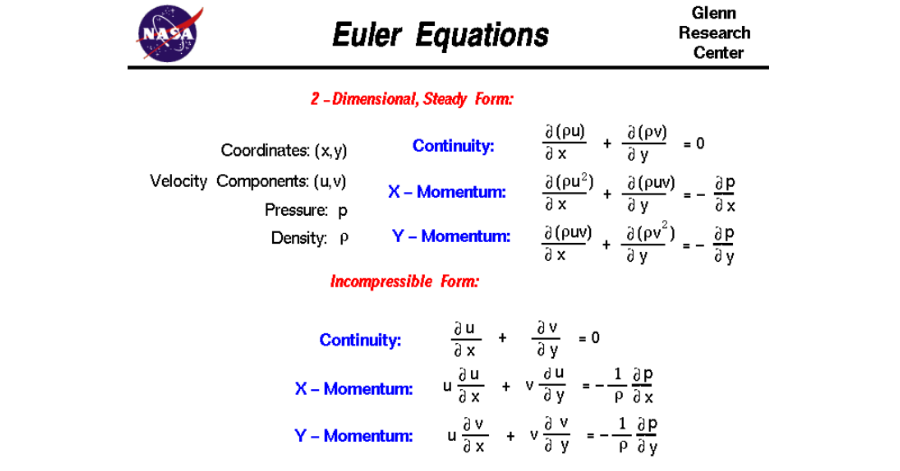
In fluid dynamics, the Euler Equations govern the motion of a compressible, inviscid fluid. They correspond to the Navier Stokes equations with zero viscosity, although they are usually written in the form shown here because this emphasizes the fact that they directly represent the conservation of mass, momentum, and energy.
Euler’s equation for a steady flow of an ideal fluid along a streamline is a relation between the velocity, pressure, and density of a moving fluid. It is based on Newton’s Second Law of Motion. The integration of the equation gives Bernoulli’s equation in the form of energy per unit weight of the following fluid.
It is based on the following assumptions:
- The fluid is non-viscous (i,e., the frictional losses are zero).
- The fluid is homogeneous and incompressible (i.e., mass density of the fluid is constant).
- The flow is continuous, steady, and along the streamline.
- The velocity of the flow is uniform over the section.
- No energy or force (except gravity and pressure forces) is involved in the flow.
Picture Source: NASA
Read more Theory Bites!